When exposing atoms to high-intensity radiation of modern short-pulse lasers, it seems expedient to regard light as a classic electromagnetic wave rather than as a stream of light particles called photons. The revolutionary concept of the photon was proposed by Einstein to explain the interaction of light and matter at low intensities. In the photon image, an atom absorbs a single photon of suitable energy to either excite, see Fig. 1a), or to be ionized (the well-known photoelectric effect). The enormous number of photons compressed in the short pulses of modern laser systems equates to enormous electric field strengths of up to 1 billion (1,000,000,000) volts per cm, compared to field strengths on the order of one volt per centimeter that occur in light sources Einstein's times were available. These high field strengths affect an atom to such an extent that ionization is close.
The inspiring story of helium atoms in strong laser fields
Fig. 1a) Stimulation of atoms at low light intensities according to Einstein: A photon (green dot) strikes an atom in its ground state and thereby instantaneously raises the electron to a higher orbit, but only if the energy of the photon is responsible for the transition need energy exactly matches.
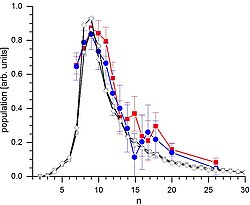
The fact that in laser fields of such high intensity, however, an excitation of atoms can be observed and explained with the classical wave pattern, has been described by H. Zimmermann et al. in a publication in the journal Physical Review Letters. In the experiment, helium atoms were exposed to the intense radiation of laser pulses with a pulse duration of 40 femtoseconds and then analyzed which states were excited in the atom, see Fig. 2. To illustrate: The laser pulse duration is one second, as the duration of a working day to the age of the universe. Although the electron in the atom is shaken vigorously during the laser pulse, the atom is not ionized, but excited. As the most important result of the study, the authors find a very good agreement of their experimental results with those of the model of the so-called "frustrated tunneling ionization" (FTI), previously described by T. Nubbemeyer et al. developed at the MBI. The FTI model is based on the image of the Starkfeldtunnelns, which was introduced about 50 years ago by the Russian physicist L. Keldysh. Thereafter, the electron can escape the binding potential of the atomic nucleus each time the laser field deforms it to such an extent that it creates a barrier through which the electron can tunnel quantum mechanically. According to Keldysh's theory, the electron moves out of the atomic network after tunneling. However, the tunneling process does not necessarily mean that the electron escapes the atom. Tunnel ionization can be suppressed ("frustrated") by closely following the movement of the electron in the combined electric field of the laser and the remaining atomic nucleus. Under certain circumstances, the electron does not receive enough energy, either from the laser field or from collisions after tunneling with the ionic core, to escape the attractive potential of the ionic trunk (see Fig. 1b).
Fig. 1b) A strong laser pulse interacting with the ground-state atom so massively affects the electron's orbit that it relaxes to a higher orbit after the laser pulse is over. The energy of a single photon does not necessarily correspond to the energy of the transition.
Through experimental confirmation by Zimmermann et al. the importance of the FTI model for the understanding of the excitation of atoms by intense laser fields is underpinned. The results illustrate that the fundamental process of excitation of atoms at the highest laser intensities can be understood as a process that combines quantum mechanical tunneling and the classical motion of the electron both in the electromagnetic laser field and in the coulomb field of the ionic fuselage.
Fig. 2) Comparison of the distribution of the principal quantum number n after excitation of He in strong laser fields: experimentally determined distribution for intensities of 1.8x1015 W/cm2 (blue dots) and 2.9x1015 W/cm2 (red squares). Calculated distribution using the FTI model for 1015W/cm2 (open diamonds) and for 1.4x1015 W/cm2 (open squares).